Time Evolution of the Expectation Value of X, and also its Fluctuation, for Phonons

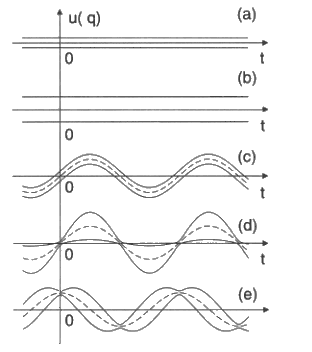
Schematic diagram of the time evolution of the expectation value and the fluctuation of the lattice amplitude operator u(±q) in different states. Here dashed lines represent the average <u(±q)>(t), while solid lines represent the envelopes <u(±q)>(t) ± (<[Du(±q)]^2>(t))^0.5 which provide the upper and lower bounds for the fluctuations in u(±q)(t).

(a) The phonon vacuum state |0>, where <u(±q)> = 0 and <[Du(±q)]^2> = 2.

(b) A phonon number state |nq, n-q>, where <u(±q)> = 0 and <[Du(±q)]^2> = 2(nq + n-q) + 2.

(c) A single-mode phonon coherent state |aq>, where <u(±q)> = 2Re(aqexp(-iwqt)) = 2|aq|coswqt, which means that aq is real, and <[Du(±q)]^2> = 2.

(d) A single-mode phonon squeezed state |aqexp(-iwqt), x(t)>, where the squeezing factor x(t) satisfies x(t) = rexp(-2iwqt). Here, <u(±q)> = 2|aq|coswqt, which means that aq is real, and its fluctuation is

    <[Du(±q)]^2> = 2[exp(-2r)cos^2wqt + exp(2r)sin^2wqt].

(e) A single-mode phonon squeezed state, as in (d). Now the expectation value of u is <u(±q)> = 2|aq|sinwqt, which means that aq is purely imaginary, and the fluctuation <[Du(±q)]^2> has the same time-dependence as in (d). Notice that the squeezing effect now appears at the times when <u(±q)> reaches its maxima while in (d) the squeezing efffect is present at the times when <u(±q)> is close to zero.

Image Source: X. Hu, Quantum Fluctuations In Condensed Matter Systems, UM Ph.D. Thesis 1997, Page 45.
    X. Hu and F. Nori, Physical Review B 53, 2419 (1996).